
How does spatial heterogeneity affect stability of trophic metacommunities?
Spatial heterogeneity of interaction strength has contrasting effects on synchrony and stability in trophic metacommunities
Abstract
Recommendation: posted 21 January 2023, validated 27 January 2023
Ulrich, W. (2023) How does spatial heterogeneity affect stability of trophic metacommunities?. Peer Community in Ecology, 100512. https://doi.org/10.24072/pci.ecology.100512
Recommendation
The temporal or spatial variability in species population sizes and interaction strength of animal and plant communities has a strong impact on aggregate community properties (for instance biomass), community composition, and species richness (Kokkoris et al. 2002). Early work on spatial and temporal variability strongly indicated that asynchronous population and environmental fluctuations tend to stabilise community structures and diversity (e.g. Holt 1984, Tilman and Pacala 1993, McCann et al. 1998, Amarasekare and Nisbet 2001). Similarly, trophic networks might be stabilised by spatial heterogeneity (Hastings 1977) and an asymmetry of energy flows along food chains (Rooney et al. 2006). The interplay between temporal, spatial, and trophic heterogeneity within the meta-community concept has got much less interest. In the recent preprint in PCI Ecology, Quévreux et al. (2023) report that Spatial heterogeneity of interaction strength has contrasting effects on synchrony and stability in trophic metacommunities. These authors rightly notice that the interplay between trophic and spatial heterogeneity might induce contrasting effects depending on the internal dynamics of the system. Their contribution builds on prior work (Quévreux et al. 2021a, b) on perturbed trophic cascades.
I found this paper particularly interesting because it is in the, now century-old, tradition to show that ecological things are not so easy. Since the 1930th, when Nicholson and Baily and others demonstrated that simple deterministic population models might generate stability and (pseudo-)chaos ecologists have realised that systems triggered by two or more independent processes might be intrinsically unpredictable and generate different outputs depending on the initial parameter settings. This resembles the three-body problem in physics. The present contribution of Quévreux et al. (2023) extends this knowledge to an example of a spatially explicit trophic model. Their main take-home message is that asymmetric energy flows in predator–prey relationships might have contrasting effects on the stability of metacommunities receiving localised perturbations. Stability is context dependent.
Of course, the work is merely a theoretical exercise using a simplistic trophic model. It demands verification with field data. Nevertheless, we might expect even stronger unpredictability in more realistic multitrophic situations. Therefore, it should be seen as a proof of concept. Remember that increasing trophic connectance tends to destabilise food webs (May 1972). In this respect, I found the final outlook to bioconservation ambitious but substantiated. Biodiversity management needs a holistic approach focusing on all aspects of ecological functioning. I would add the need to see stability and biodiversity within an evolutionary perspective.
References
Amarasekare P, Nisbet RM (2001) Spatial Heterogeneity, Source‐Sink Dynamics, and the Local Coexistence of Competing Species. The American Naturalist, 158, 572–584. https://doi.org/10.1086/323586
Hastings A (1977) Spatial heterogeneity and the stability of predator-prey systems. Theoretical Population Biology, 12, 37–48. https://doi.org/10.1016/0040-5809(77)90034-X
Holt RD (1984) Spatial Heterogeneity, Indirect Interactions, and the Coexistence of Prey Species. The American Naturalist, 124, 377–406. https://doi.org/10.1086/284280
Kokkoris GD, Jansen VAA, Loreau M, Troumbis AY (2002) Variability in interaction strength and implications for biodiversity. Journal of Animal Ecology, 71, 362–371. https://doi.org/10.1046/j.1365-2656.2002.00604.x
May RM (1972) Will a Large Complex System be Stable? Nature, 238, 413–414. https://doi.org/10.1038/238413a0
McCann K, Hastings A, Huxel GR (1998) Weak trophic interactions and the balance of nature. Nature, 395, 794–798. https://doi.org/10.1038/27427
Quévreux P, Barbier M, Loreau M (2021) Synchrony and Perturbation Transmission in Trophic Metacommunities. The American Naturalist, 197, E188–E203. https://doi.org/10.1086/714131
Quévreux P, Pigeault R, Loreau M (2021) Predator avoidance and foraging for food shape synchrony and response to perturbations in trophic metacommunities. Journal of Theoretical Biology, 528, 110836. https://doi.org/10.1016/j.jtbi.2021.110836
Quévreux P, Haegeman B, Loreau M (2023) Spatial heterogeneity of interaction strength has contrasting effects on synchrony and stability in trophic metacommunities. hal-03829838, ver. 2 peer-reviewed and recommended by Peer Community in Ecology. https://hal.science/hal-03829838
Rooney N, McCann K, Gellner G, Moore JC (2006) Structural asymmetry and the stability of diverse food webs. Nature, 442, 265–269. https://doi.org/10.1038/nature04887
Tilman D, Pacala S (1993) The maintenance of species richness in plant communities. In: Ricklefs, R.E., Schluter, D. (eds) Species Diversity in Ecological Communities: Historical and Geographical Perspectives. University of Chicago Press, pp. 13–25.
The recommender in charge of the evaluation of the article and the reviewers declared that they have no conflict of interest (as defined in the code of conduct of PCI) with the authors or with the content of the article. The authors declared that they comply with the PCI rule of having no financial conflicts of interest in relation to the content of the article.
Evaluation round #1
DOI or URL of the preprint: https://hal.archives-ouvertes.fr/hal-03829838
Version of the preprint: 1
Author's Reply, 17 Jan 2023
Decision by Werner Ulrich , posted 04 Dec 2022, validated 04 Dec 2022
, posted 04 Dec 2022, validated 04 Dec 2022
Dear Authors
I have now obtained three reviews by experts in the field. They see much value in your contribution but have also some critical comments. From my own reading i found this a well-written paper with only some minor points that have been trapped by the referees. Therefore, I invite you to resubmit a revised version of your contribution as a minor revision.
Kind regards
Werner Ulrich
Reviewed by Ludek Berec, 24 Nov 2022
Reviewed by Phillip P.A. Staniczenko, 21 Nov 2022
Review of “Spatial heterogeneity of interaction strength has contrasting effects on synchrony and stability in trophic metacommunities” by Quévreux et al.
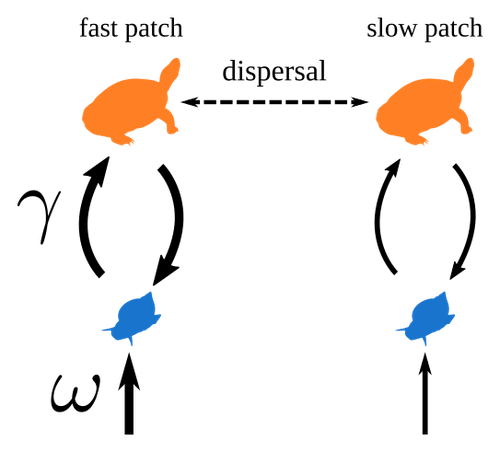
The authors consider a two-species metacommunity model comprising two resource patches and two populations of a mobile predator species that moves readily between the two patches. One resource patch is a “fast” patch in which the basal prey species is quickly replenished, while the other is a slow “patch” with lower prey growth rate; there is also asymmetry in predator-prey interaction strength between the two patches, with higher interspecific effects in the “fast” patch. The authors study the effect on population dynamics of a pulse perturbation applied to the “fast” versus “slow” patch. They find that perturbing prey abundance in the “fast” patch leads to synchrony in the dynamics of the two prey populations, whereas perturbing the “slow” patch has little effect on the correlation in dynamics between the two patches.
The manuscript is well-written, the methods appear sound, and the results are interesting. The authors do an excellent job of placing their contribution in the context of previous studies that have explored the role of interaction asymmetry on metacommunity stability. They also provide extensive supplementary information and have made R code available on GitHub. Their main result, that the properties of which patch is perturbed can have an impact on overall metacommunity dynamics, is well-argued and theoretically and practically relevant.
I have no major concerns and, below, provide a few minor comments that the authors may find helpful when revising the manuscript.
-- Introduction. I think it would be helpful to clarify for the reader how the following three terms are defined for the purposes of the study: “interaction strength,” “asymmetry of interaction strength,” and “metacommunity stability.”
-- Equation 1. It would be helpful to provide a brief explanation of the parameters and terms, e.g., what the superscripts mean in B_2^(2), and the authors could also consider annotating terms according to the familiar designations such as “predator mortality.”
-- Equations 3 and 4. It would be helpful to provide a qualitative explanation for the steps required to go from Equation 3 to Equation 4.
-- Figure 4, Panel B. I believe there is no separate plot for predator biomass CV in patch 2 because the predator populations are perfectly correlated between the two patches---nevertheless, I think it is worth reminding the reader of this point in the caption.
-- L185. The authors note that “predators have the largest total biomass.” Surely we would expect, in a two-species predator-prey system, that the total biomass of the lower trophic level, the prey, should be higher? Can the authors comment on significance of predators having higher total biomass and how much results depend on this observation?
-- L201. The authors use the term “recover” to describe a decrease in abundance of prey species following a perturbation that temporarily increases abundance. “Recover” typically describes an improvement from the perspective of the focal species, so perhaps “response” is a better, more neutral term to use.
-- Figure 5, Panel B. For the y-axis, “Direct effect,” would a log-scale be better to show more even weighting of effects < 0 compared to > 0?
-- Discussion, L219. The authors suggest that “perturbing prey in the slow patch desynchronises prey dynamics.” Personally, I’m not sure there is desynchronization taking place (i.e., change from synchrony to no synchrony), rather that the dynamics in the two patches continue to play out independently of one another.
-- Figure 6, Panel B. It would be helpful to mention in the caption the interspecific strength numbers---what they are and what they mean.
Reviewed by Diogo Provete, 02 Dec 2022
The paper by Quévreux et al. proposes a new model to understand how spatial heterogeneity promotes biomass assymetry via differential predation (variation in interaction strength) between local communities embedded in a metacommunity. This is an interesting paper that nicely fills an important gap in metacommunity theory by modeling how trophic interactions are affected by perturbations in prey populations. It's a follow up of two other papers from last year by the same authors. Since species in nature are constantly engaging in biotic interactions, a 2.0 metacommunity theory will not be achieved without understanding the role these interactions play in setting species distributions. A recent paper (Livingston et al. 2017 J Animal Ecol) has conducted an experiment to also test how predators and resource heterogeneity contribute to prey spatial dynamics. I think this is an excellent contribution, but I also have a few points to make:
1) There's key literature missing. I believe the model can dialogue with other concepts, such as the keystone community (Mouquet et al. 2012, Resetarits et al. 2018) in the sense that the 'fast' patch can act as a keystone community. These appear very briefly cited at the end of the discussion, in the context of implications for conservation. But due to the similarities in concepts, it would br good to talke about it in the introduction.
2) At the end of the introduction, in the paragraph about the movement of predators between patches, works by O Schimitz could be added, such as here and here.
Minor comments:
1) I think there's a missing word in this sentence (L. 129): "In the following, we assess the temporal variability of each population after stochastic perturbations affect the metacommunity in the vicinity of equilibrium"
2) extra "equations' in L. 132: "from the variance-covariance matrix of perturbations VE (variance-covariance matrix of E ) by solving the Lyapunov equation equation"
3) provide source for silhouettes in Fig. 1
4) I'm not sure if the subheading "Underlying mechanisms" fits in the Results, so I think you should move it to the Discussion. Some of its content is even repeated in the 1st paragraph of Discussion
5) L. 306, another good citation would be Schiesari et al. 2019
I hope authors find my comments useful.
Respectfully,
Diogo B Provete










