
Assembly in metacommunities driven by a competition-colonization tradeoff: more species in, more species out
Coexistence of many species under a random competition-colonization trade-off
Abstract
Recommendation: posted 09 August 2023, validated 10 August 2023
De Laender, F. (2023) Assembly in metacommunities driven by a competition-colonization tradeoff: more species in, more species out. Peer Community in Ecology, 100533. https://doi.org/10.24072/pci.ecology.100533
Recommendation
The output of a community model depends on how you set its parameters. Thus, analyses of specific parameter settings hardwire the results to specific ecological scenarios. Because more general answers are often of interest, one tradition is to give models a statistical treatment: one summarizes how model parameters vary across species, and then predicts how changing the summary, instead of the individual parameters themselves, would change model output. Arguably the best-known example is the work initiated by May, showing that the properties of a community matrix, encoding effects species have on each other near their equilibrium, determine stability (1,2). More recently, this statistical treatment has also been applied to one of community ecology’s more prickly and slippery subjects: community assembly, which deals with the question “Given some regional species pool, which species will be able to persist together at some local ecosystem?”. Summaries of how species grow and interact in this regional pool predict the fraction of survivors and their relative abundances, the kind of dynamics, and various kinds of stability (3,4). One common characteristic of such statistical treatments is the assumption of disorder: if species do not interact in too structured ways, simple and therefore powerful predictions ensue that often stand up to scrutiny in relatively ordered systems.
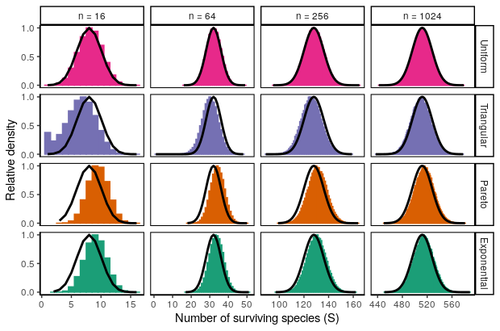
In their recent preprint, Miller, Clenet, et al. (5) subscribe to this tradition and consider tractable assembly scenarios (6) to study the outcome of assembly in a metacommunity. They recover a result of remarkable simplicity: roughly half of the species pool makes it into the final assemblage. Their vehicle is Tilman’s classic metacommunity model (7), where colonization rates are traded off with competitive ability. More precisely, in this model, one ranks species according to their colonization rate and attributes a greater competitive strength to lower-ranked species, which makes competition strictly hierarchical and thus departs from the disorder usually imposed by statistical approaches. The authors then leverage the simplicity of the species interaction network implied by this recursive setting to analytically probe how many species survive assembly. This turns out to be a fixed fraction that is distributed according to a Binomial with a mean of 0.5. While these results should not be extrapolated beyond the system at hand (4), they are important for two reasons. First, they imply that, within the framework of metacommunities driven by competition-colonization tradeoffs, richer species pools will produce richer communities: there is no upper bound on species richness, other than the one set by the raw material available for assembly. Second, this conclusion does not rely on simulation or equation solving and is, therefore, a hopeful sign of the palatability of the problem, if formalized in the right way. Their paper then shows that varying some of the settings does not change the main conclusion: changing how colonization rates distribute across species, and therefore the nature of the tradeoff, or the order with which species invade seems not to disrupt the big picture. Only when invaders are created “de novo” during assembly, a scenario akin to “de novo” mutation, a smaller fraction of species will survive assembly.
As always, logical extensions of this study involve complicating the model and then looking if the results stay on par. The manuscript cites switching to other kinds of competition-colonization tradeoffs, and the addition of spatial heterogeneity as two potential avenues for further research. While certainly of merit, alternative albeit more bumpy roads would encompass models with radically different behavior. Most notably, one wonders how priority effects would play out. The current analysis shows that different invasion orders always lead to the same final composition, and therefore the same final species richness, confirming earlier results from models with similar structures (6). In models with priority effects, different invasion orders will surely lead to different compositions at the end. However, if one only cares about how many (and not which) species survive, it is unsure how much priority effects will qualitatively affect assembly. Because priority effects are varied in their topological manifestation (8), an important first step will be to evaluate which kinds of priority effects are compliant with formal analysis.
References
1. May, R. M. (1972). Will a Large Complex System be Stable? Nature 238, 413–414. https://doi.org/10.1038/238413a0
2. Allesina, S. & Tang, S. (2015). The stability–complexity relationship at age 40: a random matrix perspective. Population Ecology, 57, 63–75. https://doi.org/10.1007/s10144-014-0471-0
3. Bunin, G. (2016). Interaction patterns and diversity in assembled ecological communities. Preprint at http://arxiv.org/abs/1607.04734.
4. Barbier, M., Arnoldi, J.-F., Bunin, G. & Loreau, M. (2018). Generic assembly patterns in complex ecological communities. Proceeding of the National Academy of Sciences, 115, 2156–2161. https://doi.org/10.1073/pnas.1710352115
5. Miller, Z. R., Clenet, M., Libera, K. D., Massol, F. & Allesina, S. (2023). Coexistence of many species under a random competition-colonization trade-off. bioRxiv 2023.03.23.533867, ver 3 peer-reviewed and recommended by PCI Ecology. https://doi.org/10.1101/2023.03.23.533867
6. Serván, C. A. & Allesina, S. (2021). Tractable models of ecological assembly. Ecology Letters, 24, 1029–1037. https://doi.org/10.1111/ele.13702
7. Tilman, D. (1994). Competition and Biodiversity in Spatially Structured Habitats. Ecology, 75, 2–16. https://doi.org/10.2307/1939377
8. Song, C., Fukami, T. & Saavedra, S. (2021). Untangling the complexity of priority effects in multispecies communities. Ecolygy Letters, 24, 2301–2313. https://doi.org/10.1111/ele.13870
The recommender in charge of the evaluation of the article and the reviewers declared that they have no conflict of interest (as defined in the code of conduct of PCI) with the authors or with the content of the article. The authors declared that they comply with the PCI rule of having no financial conflicts of interest in relation to the content of the article.
Z.R.M. was supported by the National Science Foundation Graduate Research Fellowship Program (DGE-1746045). M.C. and F.M. are supported by the CNRS 80 prime project KARATE
Evaluation round #1
DOI or URL of the preprint: https://doi.org/10.1101/2023.03.23.533867
Version of the preprint: 1
Author's Reply, 13 Jul 2023
Decision by Frederik De Laender, posted 23 May 2023, validated 25 May 2023
Both reviewers highlight the merits of the presented work, but also list a number of suggestions to strengthen the paper. These revolve around two aspects: (1) improving the link between the presented results and empirical systems; (2) explaining how and why the presented results differ from (or confirm) those already available in the published literature. I agree with these suggestions, and personally find the second aspect most important.
Reviewed by anonymous reviewer 1, 02 May 2023
In their research, Miller et al. explored the coexistence of various species in a random metacommunity with a competition-colonization trade-off. Their findings revealed that, across a broad range of random setups, the number of surviving species followed a binomial distribution mean of half the original species pool size. These results are novel and add to the growing interest in understanding what occurs when a community is unstable, rather than solely determining if it is stable or not. The study is well-written, although it may not be easily accessible to empirical researchers. I checked the derivations in the main text (not the SI) and they all seem solid. I congratulate the authors for this nice work. I do not have any major concerns. However, I believe it would be beneficial if the authors could establish a stronger connection between this work and existing literature. I have included more detailed comments below.
1. Spacing of colonization rates (Figure 4). I was a bit surprised that the colonization rates of surviving species mimic the original distribution. This relates to the work by Scheffer et al. on emergent neutrality (10.1073/pnas.0508024103) where they found the initial niche spacing and the survived quite different. What drives the fundamental difference between these results? To be clear, I am not asking for a detailed comparison; some speculations are sufficient, although analytic arguments would be great.
2. Unlimited species richness (Figure 2). I am curious if the unlimited species richness is likely to be stably maintained regarding parameter perturbation. As a context, this result reminds me of another study showing unlimited richness can be supported with facilitation among competitors (10.1111/j.1461-0248.2008.01204.x), however, later studies have found it increasingly less robust to parameter perturbation (10.1111/ele.12350). I think this problem applies to many other models allowing infinite species richness. Of course, the model setups between that model and this paper are quite different, but I am curious if the same issue occurs here. If so, perhaps this is at least worth highlighting in the discussion.
3. I am also curious about the statement that infinite re-invasions one-at-a-time would lead to the same outcome as invasion all at once (L111-122). This result is not generally true with priority effects (10.1111/ele.13870), for example with the existence of a compositional cycle. I guess that this result relies on the special structure of GLV model imposed by Eq. 3, which seems similar to the setup of Servan et al. I am curious whether the key results (B(n, 1/2) and assembly equivalence) fundamentally rely on this property, or they can be generalized in more general GLV structures.
Minor comments:
1. Figure resolutions are low.
2. I am wondering if the results would remain consistent if the colonization rates were obtained from a mixed distribution. For instance, if there were an equal likelihood of the colonization rate being sampled from an exponential distribution or a uniform distribution. If this were true, this would further strengthen the argument in the paper about the universality of the results. To be clear, I am not seeking an analytical outcome; simulation results would suffice.
https://doi.org/10.24072/pci.ecology.100533.rev11Reviewed by Canan Karakoç, 11 May 2023
The manuscript “Coexistence of many species under a random competition-colonization trade-off” explores the coexistence under competition-colonization trade-off using a slightly different and more realistic approach. It employs randomly assembled communities and a probabilistic approach rather than a traditional C-C model. Moreover, the manuscript explores the outcomes when species are introduced one at a time or through de novo speciation. Results highlight that rich communities might arise through ecological assembly under competition colonization trade-off. The question is interesting and timely, theoretical approach is different than classical CC models, which adds value for further use of the methods. Results are well communicated and discussed.
General comments:
The manuscript could be more convincing in that the randomly assembled communities are closer to the real world than the models with fine-tuned species traits. For example, how would the approach be challenged with parameterization using real species traits? More biological/empirical support might be needed to justify the rationale behind the framework chosen. What could be the biological reason that the niche shadow reduces with colonization, for instance?
Historical contingency has been briefly mentioned but not elaborated on. For example, are there priory effects when the species are introduced in a one-at-a-time manner? Another issue is the increased diversity after speciation effects. In a real-world example, generation times would be important for the speciation. How this time to speciate issue was handled is not very clear.
Specific comments:
Title: I wonder if the title would be more appealing if it mentions “randomly assembled communities” and the probabilistic estimations.
Abstract: The species parameters are clearly defined here. If the manuscript wants to use traits instead of parameters, maybe can define it so with the parameters. it will read better. Exclusion (shadow) is an important part of the manuscript. The abstract can briefly mention that dependency.
Line 51: Maybe highlight the two important results.
Lines: 71-72: The manuscript could explain the possible ecological reason for distribution selection.
Lines 82-83: Maybe a couple of references for coexistence close to n and exclusion close to 0 are theoretically almost impossible.
Line 192-195: The manuscript can mention some other trade-off scenarios.
Line 233-234: Although c is always higher than m, experiencing the same mortality rate for all species is a sort of environmental filtering. Justify if it is not the case.
Eq.s 4-5: Is there ecological reasoning that the niche shadow decreases with increasing c?
Eq.s 7-8: I wonder if correspondence between random variables K and the final coexisting species can be verbalized in the results with a sentence or two.
Figure 1: is there a way to illustrate that the niche shadow of a species reduces when affected by another species?
Figure 4: I think the spacing between rates could be explained better for a broad audience.
Lines 161-162: It is hard to see connect niche partitioning phenomena to the theoretical results.
https://doi.org/10.24072/pci.ecology.100533.rev12









