

 and Kevin Shear McCann based on reviews by Frédéric Barraquand and 1 anonymous reviewer
and Kevin Shear McCann based on reviews by Frédéric Barraquand and 1 anonymous reviewer
In his 1972 paper “Will a Large Complex System Be Stable?” [1], May challenges the idea that large communities are more stable than small ones. This was the beginning of a fundamental debate that still structures an entire research area in ecology: the diversity-stability debate [2]. The most salient strength of May’s work was to use a mathematical argument to refute an idea based on the observations that simple communities are less stable than large ones. Using the formalism of dynamical systems and a major results on the distribution of the eigen values for random matrices, May demonstrated that the addition of random interactions destabilizes ecological communities and thus, rich communities with a higher number of interactions should be less stable. But May also noted that his mathematical argument holds true only if ecological interactions are randomly distributed and thus concluded that this must not be true! This is how the contradiction between mathematics and empirical observations led to new developments in the study of ecological networks.
Since 1972, the theoretical corpus of ecology has advanced, building on the formalism of dynamical systems, ecologists have revealed that ecological interactions are indeed not randomly distributed [3,4], but general rules are still missing and we are far from understanding what determine the exact network topology of a given community. One promising avenue is to understand the relationship between different facets of the concept of stability [5,6]. Indeed, the classical approach to determine whether a system is stable is qualitative: if a system returns to its equilibrium when it is slightly moved away from it, then the system is considered stable. But there are several other aspects that are worth scrutinizing. For instance, when a system returns to its equilibrium, one can characterize the corresponding transient dynamics [7,8], that is asking fundamental questions such as: what is the trajectory of return? How long does it take to return to the equilibrium? Another fundamental question is whether the system remains qualitatively stable when the distributions of interactions strengths change? From a biological standpoint, all of these questions matter as all these aspects of stability may partially explain the actual structure of ecological networks, and hence, frameworks that integrate several facets of stability are much needed.
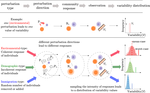
The study by Arnoldi et al. [9] is a significant step towards such a framework. The strength of their formalism is threefold. First, instead of considering separately the system and its perturbations, they considering the fluctuations of a perturbed ecological systems and thus, perturbations are parts of the ecological system. Second, they use of a broad definition of perturbation that encompasses the types of perturbations (whether the individual respond synchronously or not), their intensity and their direction (how the perturbations are correlated across species). Third, they quantify the instability of the system using variability which integrates the consequences of perturbations over the whole set of species of a community: such a measure is comparable across communities and accounts for the trivial effect of the perturbations on the system dynamics.
Using this framework, the authors show that interactions within a stable community leads to a general relationship between variability and the abundance of individually perturbed species: if individuals of species respond in synchrony to a perturbation, then the more abundant the species perturbed the higher the variability of the system, but the relationship is reverse when individual respond asynchronously. A direct implications of these results for the classical debate is that the diversity-stability relationship is negative for the former type of perturbations (as in May’s seminal paper) but positive for the latter type. Hence, the rigorous work of Arnoldi and colleagues sheds a new light upon the classical debate: the nature of the perturbation regime prevailing within a community affects the slope of the diversity-stability relationships and given the vast diversity of ecological communities, this may very well be one of the reasons why the debate still endures.
From a historical perspective, it is interesting that ecologists have gone from looking at random webs to structured webs and now, in a sense, Arnoldi et al. are unpacking the role of differentially structured perturbations. The work they achieved will doubtlessly be followed by further theoretical investigations. One natural research avenue is to revisit the role of the topology of ecological networks with this framework: how the distribution of interactions and their strength affect the general relationship they unravel? Finally, this study demonstrate that the impact of the abundance of a species on the variability of the system depends on the nature of the perturbation regime and so the distribution of species abundances within a community should be determined by the prevailing perturbation regime which is a prediction that remains to be tested.
References
[1] May, Robert M (1972). Will a Large Complex System Be Stable? Nature 238, 413–414. doi: 10.1038/238413a0
[2] McCann, Kevin Shear (2000). The Diversity–Stability Debate. Nature 405, 228–233. doi: 10.1038/35012234
[3] Rooney, Neil, Kevin McCann, Gabriel Gellner, and John C. Moore (2006). Structural Asymmetry and the Stability of Diverse Food Webs. Nature 442, 265–269. doi: 10.1038/nature04887
[4] Jacquet, Claire, Charlotte Moritz, Lyne Morissette, Pierre Legagneux, François Massol, Philippe Archambault, and Dominique Gravel (2016). No Complexity–Stability Relationship in Empirical Ecosystems. Nature Communications 7, 12573. doi: 10.1038/ncomms12573
[5] Donohue, Ian, Helmut Hillebrand, José M. Montoya, Owen L. Petchey, Stuart L. Pimm, Mike S. Fowler, Kevin Healy, et al. (2016). Navigating the Complexity of Ecological Stability. Ecology Letters 19, 1172–1185. doi: 10.1111/ele.12648
[6] Arnoldi, Jean-François, and Bart Haegeman (2016). Unifying Dynamical and Structural Stability of Equilibria. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Science 472, 20150874. doi: 10.1098/rspa.2015.0874
[7] Caswell, Hal, and Michael G. Neubert (2005). Reactivity and Transient Dynamics of Discrete-Time Ecological Systems. Journal of Difference Equations and Applications 11, 295–310. doi: 10.1080/10236190412331335382
[8] Arnoldi, J-F., M. Loreau, and B. Haegeman (2016). Resilience, Reactivity and Variability: A Mathematical Comparison of Ecological Stability Measures. Journal of Theoretical Biology 389, 47–59. doi: 10.1016/j.jtbi.2015.10.012
[9] Arnoldi, Jean-Francois, Michel Loreau, and Bart Haegeman. (2019). The Inherent Multidimensionality of Temporal Variability: How Common and Rare Species Shape Stability Patterns.” BioRxiv, 431296, ver. 3 peer-reviewed and recommended by PCI Ecology. doi: 10.1101/431296
DOI or URL of the preprint: https://doi.org/10.1101/431296
Version of the preprint: 2
Dear Editors,
Please find below the list of modifications to address the remaining comments. We have also modified the layout of the preprint (PCI front page and then two columns -see document attached, which is slightly different to the most recent bioRxiv upload, which we will upload asap). Thank you again for your investment in our paper.
With best regards,
Jean-Francois Arnoldi, Michel Loreau and Bart Haegeman
 and Kevin Shear McCann, posted 13 Mar 2019
and Kevin Shear McCann, posted 13 Mar 2019Dear Dr. Arnoldi,
We are glad to inform you that we have decided to recommend your preprint and that we are currently editing our recommendation. In the meanwhile, we ask you to address the minor comments we listed below (note that line numbers below refers to the preprint available on biorxiv). Once the new version uploaded, we will post our recommendation on PCI.
Dr. Kevin Cazelles and Pr. Kevin S. McCann
MINOR COMMENTS:
There is currently no mention about how the topology of the network may affect your results. This may surprise some readers and it may be worth mentioning explicitly that you did not explore this in this study.
l. 155: we are unsure that the use of “vs” in this subtitle is justified.
l.172, in the main text, $\sigma_{out}$ is never defined for more than one species, we think it would be relevant to add a link to the the relevant equation in the Appendix (B4).
l.185-186: This sentence is very important and we think it is worth adding the notations defined above, so instead of:
“Once intensity is controlled for, perturbations can still differ in how their intensity is distributed and correlated across species.”
We suggest (if, we are correct):
Once perturbation intensity (\sigmain) is controlled for, perturbations can still differ in how their intensity (\sigmai) is distributed and correlated in time across species ($\cor(\xii,\xij)$).
Caption of figure 2:
In the text the number of species using for simulation is either 40 or 50, which is the correct one?
Figures 4 and 5: We suggest to add the symbol of the variability in the y-axis label “Induced variability ($\mathcal_{V}$)” so the reader can quickly recall what you are referring to.
Figure 6: Same as above, we recommend “Invariability ($\mathcal{I}$)” as y-axis label and it might be worth mentioning that it is a measure of stability in the caption.
It might be helpful for the reader to stress out that Figures 4 and 5 correspond to a single community but that the results are general.
For Figure 5, you should mention explicitly the quantitative difference between weak and strong interactions.
l.318-325: This paragraph is quite dense and it is hard to follow, it is crucial to explain to the reader how to read May’s work in the light of your conceptual framework. Also it might be worth explaining (or citing a paper dealing with this topic) why when the number of interactions increase the number of rare species increases.
l. 376: “species ability to buffer exogenous perturbations is inversely proportional to their abundance”. This sentence is very helpful and so it might be worth adding it in the abstract. Also, would it be more accurate to write “inversely proportional to the square root of their abundance”?
Additional requirements of the managing board :
When preparing the final version of your preprint, and in order to reach a better referencing and greater visibility of your recommended preprint, could you please do the following modifications :
=> First modification:
(i) add the following sentence in the acknowledgements: "This preprint has been reviewed and recommended by Peer Community In Ecology (https://dx.doi.org/10.24072/pci.ecology.100017) »
Doing so is very important because it would:
-indicate to readers that, unlike many other preprint in this server, your pre-print has been peer-reviewed and recommended
-make visible this information in Google Scholar search (which is quite important).
==> Second modification: In the 'How does it work?’ section and in the code of conduct, you can read that
-Data must be available to readers after recommendation, either in the text or through an open data repository such as Zenodo (free), Dryad (to pay) or some other institutional repository. Data must be reusable, thus metadata or accompanying text must carefully describe the data.
-Details on quantitative analyses (e.g., data treatment and statistical scripts in R, bioinformatic pipeline scripts, etc.) and details concerning simulations (scripts, codes) must be available to readers in the text, as appendices, or through an open data repository, such as Zenodo, Dryad or some other institutional repository. The scripts or codes must be carefully described so that they can be reused.
-Details on experimental procedures must be available to readers in the text or as appendices.
-Authors must have no financial conflict of interest relating to the article. The article must contain a "Conflict of interest disclosure" paragraph before the reference section containing this sentence: "The authors of this preprint declare that they have no financial conflict of interest with the content of this article."
This disclosure may be completed by a sentence indicating that some of the authors are PCI recommenders: “Bart HAEGEMAN is one of the PCI Ecology recommenders.”
==> Third modification: In addition, we suggest you to remove line numbering from the preprint.
==> Fourth, (if you wish) we advise you to use templates (word docx template and a latex template) to format your preprint in a PCI style . This is optional. Here is the links of the templates:
https://peercommunityin.org/templates/
DOI or URL of the preprint: 10.1101/431296
Version of the preprint: 1
Dear Editors,
We have now revised our manuscript in which we have addressed all comments by the editors and reviewers.
Thanks to the constructive and thorough work by editors and reviewers, we believe the article to be much more focused and clear. In particular, following the criticism of Frédéric Baraquant concerning the use of the word "spectrum", we changed the title of our manuscript to: "The inherent multidimensionality of temporal variability: How common and rare species shape stability patterns".
Although the manuscript length has not changed significantly, we made substantial efforts to focus our message and shortened the discussion section to make it more factual and less speculative. Our paper is now organised in two parts. The first establishes a general theory for variability, emphasizing its highly multidimensional nature, while the second part explains that a generic variability-abundance pattern can emerge in species-rich communities. The relevance of this pattern is illustrated by explaining that it can shape diversity-stability relationships.
This new version has been uploaded on BioRxiv, as a revision of the previous preprint (with Matlab code as supplementary material). Attached is a detailed response to all comments as well as a comparison file that highlights the (numerous) changes that we have made to the text.
We hope that you will find the revised manuscript suitable for recommendation by PCI Ecology.
With best regards,
Jean-François Arnoldi, Michel Loreau and Bart Haegeman
 and Kevin Shear McCann, posted 13 Nov 2018
and Kevin Shear McCann, posted 13 Nov 2018Dear Dr. Arnoldi,
Thank you for submitting your preprint "The variability spectrum of ecological communities: How common and rare species shape stability patterns" to PCI Ecology. We have now received two reviews of your manuscript.
Both reviewers regard your work as a strong contribution to the understanding to the diversity-stability debate. We agree with the reviewers, it is indeed an insightful study that rigorously demonstrate that the nature of the perturbation matters, in the sense
that it can dramatically changes the relationship between diversity and stability.
We would therefore be happy to recommend it once the reviewers' comments are addressed.
Most reviewers comments are suggestions that will doubtlessly improve the overall clarity of the manuscript and so we regard the revision required as a minor one even though we acknowledge that the edition of a long paper could be time-consuming. One of the reviewer has provided a very detailed list of comments that should help you targeting parts that currently miss clarity. We would also like to highlight some of the reviewers' comments and expand on them:
One reviewer points out that the paper is fairly long and that there is enough material for more than one paper. We agree. That said, we would be happy to recommend an even longer preprint that have a better balance between accuracy and pedagogy. The length issue may be one you will be dealing with depending on were you submit your manuscript, not an issue for a recommendation for PCI (as far as we understand the rules).
So far, the reader cannot reproduce the study, it is very important that the manuscript includes all the simulations details so the reader can reproduce your analysis. Note that one way to deal with this comment is to share your code.
One reviewer mentions that you must differentiate previous mathematical findings from new ones. We agree, this would help understanding how to obtain equation B1. Another thought we had while reading the appendix is that you should consider adding a reference that introduces Stochastic Differential Equations to help readers not familiar with this to have a better understanding of the approach you developed.
Finally, we would like to add a few comments as well as two typos:
Circularity: Basically, the authors use a model where the higher the value of $\alpha$, the more density dependent the perturbations are. Based on it, they found that the variability response for low values of $\alpha$ is driven by rare species whereas common species drive the response when $\alpha$ is high. Isn't it something expected? We think the authors should expand on this. This is not a problem but as written, it feels a little hidden behind the types of perturbations (demographic, immigration etc.)
Figures 4-6 examine slopes for different values of $\alpha$. why not report the values of the slope along a gradient of $\alpha$ value
There are several notations use throughout the appendix, most of them are common some other are introduces by the authors, so we would suggest that you add a table of notations when the set of appendix are introduced p 35.
May be a naive one: in appendix A, why not saying that $\mathbf{u}k$ follows a multinormal distribution $\mathcal{N}(\mathbf{0},Cu)$?
$C_x$ instead of C in equation (A7)
l. 287: We think it should be "5th to 95th" instead of "10th to 90th".
We now invite you to respond to the reviewers' comments as well as the few comments we added and submit a revised manuscript for a potential recommendation by PCI.
Sincerely,
Dr Kevin Cazelles and Pr Kevin S. McCann
In this theoretical study Arnoldi et al. investigate how the variability of community dynamics is affected by different types of perturbations. The stability/variability of ecological communities is set in relation to species´ equilibrium biomasses for different perturbation scenarios, and different abundance classes’ roles in governing stability are acknowledged. Overall, the authors conclude that a multidimensional view on stability allows one to better appreciate the dynamical richness of ecological communities.
In general, I think this is a very strong and well conducted study. Adding a stochastic component into the thinking about complex ecological systems is interesting and much needed. However, I have a few points that should be addresses before recommending this study.
Major point: I miss an important reference to a similar study (Ives et al. 2003; Ecological Monographs). The similarity between this study and Ives et al. should specifically be addressed and discussed. Both studies compare the (output) variability of the stationary distribution to the (input) variability of process errors, in ecological models. Yet, Ives et al. uses linear discrete models.
Minor comments: It would be interesting to see how the mean and sd of equilibrium biomasses change as diversity increase in the networks. I would think that eq. biomasses decrease as diversity increase, thus affecting the perturbation magnitudes.
It would be interesting to see how the two parts of stability, i.e. varout & varin, change when diversity in the communities change (i.e. producing figures similar to fig. 6). This would add valuable information about what is contributing the most to stability. I would suspect that var_in is highest for the environmental type of perturbation, followed by demographic and immigration types of perturbations.
I agree that it is important to point out that different types of perturbations produce different stability patterns. Yet, since the stability measures presented here appear to be linearly related to each other (at least the mean responses), I would argue that a single measure of stability would hold a great deal of information about the stability of the whole community. For example, asymptotic resilience is linearly and negatively related to the mean stability for each perturbation scenario presented here (yet with different slopes; Fig. 6). Thus, a more simple one-dimensional representation of stability may be enough when calculating the stability properties of a community. Please comment on this!
https://doi.org/10.24072/pci.ecology.100030.rev11 , 08 Nov 2018
, 08 Nov 2018